Where have the algorithms gone? The Australian Curriculum Review: Mathematics

ACARA has released its draft revision of the Australian Curriculum: Mathematics with the view to “refine, realign and declutter” the content. This has been long overdue. Since the curriculum was first published in 2010, Australia has performed poorly on international tests in mathematics. There is a lot to like about the revision. Simply splitting the three strands into the traditional six is a big plus! More detail such as this serves to provide clarity.
Many positives can be taken from this revision. Upon a careful review of the proposed F-6 content, the following three changes are most worthy of praise.
Firstly, there is certainly far greater clarity in the content descriptions. Many of the wide-sweeping statements of the original curriculum that were left open to interpretation have been replaced with much more detail. For example, the original content description in Year 4 below is quite vague and raises several questions. What fractions should be addressed? How important is context? How should equivalent fractions be modelled? The proposed change answers these questions by stipulating the fractions to be explored, and by replacing context with the greater need for representations – of which ‘symbolic notation’ is assumed to be only one.
| Original | Investigate equivalent fractions used in contexts (ACMNA077) |
|
Proposed |
Recognise the relationships between families of fractions (halves, quarters and eighths; fifths and tenths; thirds, sixths and twelfths) including equivalence. Use different representations (including fraction notation) to designate parts of a whole (AC9M4N05) |
Secondly, there also seems to be better content flow across year levels. The format of this revision allows the reader to see learning trajectories across grades. This in itself is informative and particularly helpful for the hundreds of schools that serve multi-age classrooms. This excerpt (below) from the proposed scope and sequence for SPACE shows a consistent flow of topics across Foundation to Year 4. The first row addresses shapes and objects. The second row deals with location and position and it is clear that transformations that lead to symmetry, which is described in the third row, is first introduced in Year 2. In our experience, the lack of careful sequencing has been a major pain point for teachers. This revision will improve the ability of teachers to make good decisions while greatly reducing the time required for planning.
| Year F | Year 1 | Year 2 | Year 3 | Year 4 |
|
sort, name and make familiar shapes and objects. Recognise and describe familiar shapes and objects within the environment using everyday language (AC9MFSP01) |
recognise, compare and classify familiar shapes and objects in the environment, using obvious features. Identify the similarities and differences between them (AC9M1SP01) |
recognise, compare and classify regular and irregular shapes and objects describing features and properties using spatial terms (including parallel sides) (AC9M2SP01) | analyse, classify and make models of objects, identifying key features and explaining why these features make them suited to their uses (AC9M3SP01) |
use combinations of shapes and objects to make or approximate more complex shapes and objects in the environment (AC9M4SP01) |
| describe position and movement of self and objects in relation to other objects and locations within a familiar space (AC9MFSP02) |
give and follow directions to move people and objects to different locations (AC9M1SP02) |
locate positions and identify relative positions of key features of a familiar space represented in two-dimensions. Move positions following directions and pathways (AC9M2SP02) | create, use and interpret models of familiar environments positioning representations of key landmarks and objects relative to each other (AC9M3SP02) | create and interpret grid maps using grid references and directions to locate and describe positions and pathways (AC9M4SP02) |
| recognise and explain the effect of one-step transformations (including translation, reflection and rotation) on shapes using dynamic geometric software where appropriate (AC9M2SP03) |
identify line symmetry in the environment, using terms such as vertical, horizontal and diagonal to describe the lines (AC9M3SP03) |
recognise rotational symmetry of shapes and create symmetrical patterns, and pictures using dynamic geometric software where appropriate (AC9M4SP03) |
(ACARA. Australian Curriculum: Mathematics – Scope and Sequence F – 6.
Consultation Curriculum. p. 2&5)
Finally, the biggest improvement of the Australian Curriculum is the introduction of computational thinking. This is the content that computer scientists suggest should be taught to equip students with the skills needed for today’s jobs and the jobs of the future.
It is generally accepted that there are four key aspects of computational thinking as shown in this diagram, which begins in a search for patterns and culminates in the writing of an algorithm or the code for a computer to follow.
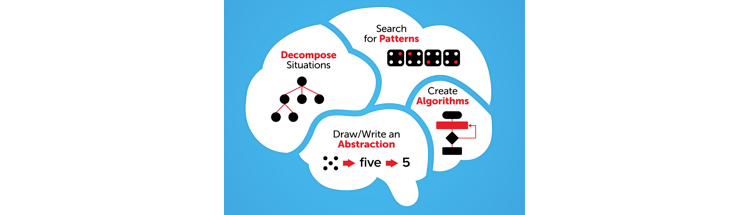
Notably, the international PISA tests in mathematics will include this content in all future tests and tasks.
The revision now includes computational thinking in the Algebra strand of Years 3–6; the Space strand of Years 5–6, and in Probability in Year 6. It should be mentioned that in the past, algorithms are generally referred to those step-by-step written procedures that students recall from memory and follow to get an answer. Interestingly, the word ‘algorithm’ does not appear in the Number Strand of the revised Australian curriculum. It is only used with Computation Thinking which is about ‘creating’ algorithms rather than recalling them – bravo!
As this is a major shift in mathematics instruction, we expect that many teachers may not understand the meaning of the content descriptions specific to computational thinking and therefore, also their intent. No doubt there will be much professional learning required to support teachers as they implement this revised curriculum. In short, you cannot have success with one and not the other. ORIGO Education has spent the last two years pioneering the inclusion of computational thinking to primary school instruction. In doing so, we have been conducting professional learning workshops specific to computational thinking for local and international F-6 teachers. In fact, it is one of the major topics that will be addressed in our inaugural ORIGO Academy of Mathematics Education that is being offered in person in Brisbane later this year. For more information, visit https://www.origoeducation.com.au/origo-academy-of-mathematics-education/